TRIGONOMETRIC EQUATIONS Solving Equations (Page 8 of 11)

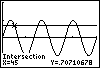
Now we will find the second intersection point (from the first cycle). We will use a guess of 40.

 2nd CALC key. 5: menu selection.
 ENTER key. (Mark the first graph)
 ENTER key. (Mark the second graph)
 40 ENTER key.		 Calculator screen image.

The intersection point is approximately (45, 0.707). The x-coordinate of this point, x = 45, is also a solution to the equation. The solutions from the first cycle are {15° , 45°}. All other solutions will occur on a different cycle. Since the period of this function is 360°/3 = 120°, the other solutions will be separated by a multiple of 120°. So the solution set of the equation, in degrees, is {15deg+-120deg*n, 45deg+-120deg*n}, where n is any integer.

One advantage of degree mode is the ability to recognize the resulting angles that are solutions to the equation. If radian mode is used, the results will usually be numbers involving π which are much less familiar in decimal form.
Copyright © 2010 Turner Educational Publishing
Go back to the previous page. Go to the next page. Go to the Table of Contents. Go to the Index page. Go to the Quiz page. Exit to the Home page.